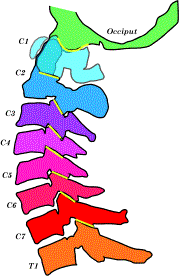
Spinal Dynamics II: Lower Cervical Spine Anatomy Anatomy of the Lower Cervical SpineThe caudal part of the axis and the rest of the cervical vertebrae have a different anatomy than the upper cervical assemblage. They are all quite similar in their structure, at least on the level at which we will be considering them here (Langer 2005q). The vertebral body is cylindrical with lateral flanges (uncinate processes) that cup the superjacent vertebral body and restrict its movements and facets that are inclined about 45¡ to the coronal plane of the vertebra, which also restrict the possible movements. The net effect of this architecture is that movements between the vertebrae are either around an oblique axis tilted about 45¡ relative to the vertebral body or sagittal, about a transverse axis located in the subjacent vertebral body. This anatomy makes the cervical spine an interesting region to model with quaternions and to study movements in an assemblage of concatenated bones.
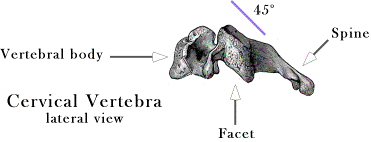
A typical cervical vertebra, viewed from the left side, showing the inclination of the facet surfaces.
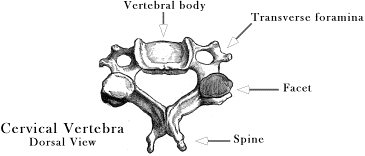
A typical cervical vertebra viewed from above and behind, showing the relative positions of the vertebral body, the articular pillar, and the transverse foraminae.
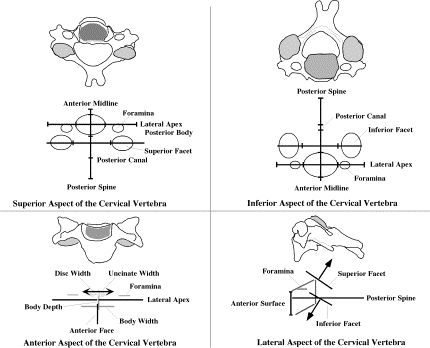
The summary measurements from a collection of cervical vertebrae are illustrated with both drawings and schematics for a number of views of a cervical vertebra. While there are differences between the individual cervical vertebrae in different necks and in the same neck, they are similar in certain respects that are important in modeling movements (Langer 2005q; Langer 2005r; Langer 2005s). We will not go into detail here about the anatomy of an archetypical cervical vertebra. The essential elements for present purposes is that the vertebral bodies are cylindrical with a modest amount of tapering from front to back, so that they stack in a convex arch that bulges anteriorly. They are broader (from side to side), than they are deep (from front to back), and deeper than they are tall (from top to bottom). They have an articular pillar that lies posterior and lateral to the vertebral body, with tilted facets that are consistently occupy the nearly the same plane on both sides, a plane that is tilted about 45¡ forward relative to the coronal plane of the vertebra. Movements of Lower Cervical VertebraeThe common inclination of the facet planes means that lateral movements may occur about an axis in that plane and in a midsagittal plane perpendicular to that plane. However, the uncinate processes of the vertebral body, together with the intervertebral disc restrict the axis of rotation to the intersection of those two planes, a tilted sagittal axis of rotation in the midline of the plane of the facets. It is interesting that the axis of rotation is in the same direction as the long axis of the vertebral spine. In this movement, the superjacent facet slides rostrally on one side and caudally on the other side.
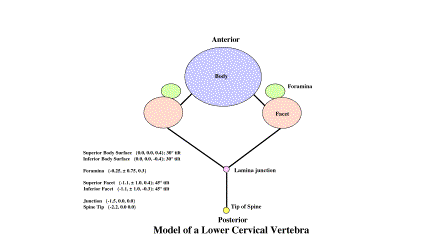
By the comparison of several cervical vertebrae from different levels of the cervical spine a representative schematic has been constructed. The schematic for a representative cervical vertebra that was used for computation is shown here. Movements may also occur about a second axis of rotation. Rotational movement can occur about a transverse axis, through the vertebral body. The superjacent vertebral body rocks forward over the subjacent vertebral body. In that type of movement the inferior facets of the superjacent vertebra side up or down over the superior facets of the subjacent vertebra. In a flexed position the superjacent facets sit rostral and ventral relative to the subjacent facets. Experimental measurements of instantaneous axes of rotation have been done and we can use them to choose appropriate locations for the axes of rotation (Kapandji 1974; Nordin and Frankel 1989; Bogduk 1999; Bogduk and Mercer 2000). There is not a single of instantaneous axis of rotation for the whole movement. The axis probably shifts as the movement progresses, but the shift is not large relative to the size of a vertebrae. Also the placement of the axis of rotation varies with the joint is being considered. However, the axis of rotation is generally through the center of the subjacent vertebral body. For present purposes, the details are not critical, since we are primarily interested in the general principles of the movements and how the movements interact. In order to make sense of the movements of the lower cervical spine, it is necessary to reduce the number of varied parameters to as small a set as possible. For that reason we are assuming that all the vertebrae are essentially the same although there are clearly substantial systematic differences between the vertebrae in all necks and between necks. However, as indicated the differences are largely in attributes other than the articulations, so they may affect muscle actions, but are less apt to affect the nature of the movements between vertebrae. Once we understand the general principles, it will be possible to address the consequences of reduced or altered motion in a segment and particular muscle actions.
A series of sagittal sections of a cervical spine have been analyzed and condensed into a single image that summarizes the shapes and spatial relations between the cervical vertebrae. As with any particular neck, there are deviations from uniformity, but the basic configuration is similar to the images produced by the mathematical model (see below). A second source of information is the articulated spine, particularly the spine in alive individuals. To that end a number of examples of x-rays, CT scans, MRI, and dissections of frozen specimens were analyzed to determine how the vertebrae are articulated. The above figure was taken from a serially sectioned neck, where one could unambiguously identify the locations of the vertebral components, but it is most respects similar to images collected from a number of living individuals. Building a Cervical SpineStarting with the anatomy of a prototypical lower cervical vertebra and the relations between cervical vertebrae, one can begin to build a model of the spine that will allow one to ask questions about the manner in which the cervical spine moves. The starting point is to examine individual vertebrae and form a schematic description. As stated above, although the cervical vertebrae clearly differ from each other, they also share a great deal of their basic anatomy. That is especially true of the anatomy that relates to the movements in the cervical spine. Because of the tapering of the vertebral bodies and the intervertebral discs, the cervical spine is arched so that each vertebral body is tilted on average about 10¡ posterior relative to the vertebra caudal to it. In actual necks there may be greater or lesser inclinations between adjacent vertebrae, but there is no consistent pattern to the deviations. The differences are probably individual and may vary with the posture assumed by the individual being imaged. The model is developed in detail elsewhere ( Once again, we assume a standard relationship, although the model does allow one to write different framed vectors and rotation quaternions for each vertebra. However, introducing those types of variations at the outset only confuses the analysis, because we do not know what is due to the basic organization of cervical vertebrae and what is due to individual variation. The main variation used is allowing different amounts of rotation in different joints. That is because experimental studies indicate that there is substantially more movement between the middle vertebrae (~10¡) than between the highest and lowest cervical vertebrae (~5¡).
The model of the lower cervical spine represents the cervical vertebrae as flattened oblong tori for the vertebral body and flattened discs for the facets. In this instance the neck is represented in neutral position. The gaps in the tori mark the vertical and horizontal meridians of the vertebra. When we build a neck that is uniform in the spatial relations between its vertebrae, the artificial neck looks reasonably like a natural neck. In the above figure, the vertebrae are represented by fat tori that have been constructed to have the correct ratio of height to depth to width. The intervertebral discs are not drawn, but they are part of the calculation and the distances between the tori are appropriate for a young healthy cervical spinal column. Flattened discs match the location, size, and orientation of the facet joints. The discs have been drawn so that the distance between them corresponds to the distance between bony profiles of the facets joints in lateral x-rays. The discs are useful to visualize the relationships between the facets as the spine moves. They are frequently not s drawn in the output images, especially if they are not relevant to the question under consideration.
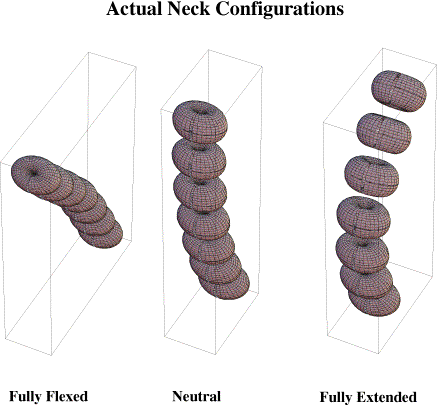
The neck is represented in three positions that approximate actual neck configurations. The facet discs have not been included in these examples. The central neck is in neutral position and the same neck is re-computed using representative flexion and extension rotations in the intervertebral joints. Watching It MoveEven though we assume that all the vertebrae are morphologically similar with comparably situated axes of rotation, each vertebra experiences a different movement when the vertebrae move. The excursion that a rostral vertebra experiences as the neck moves in all of its joints may be quite complex. Fortunately, such relationships are built into the model when we describe the anatomy of a standard vertebra and write the axes of rotation that link pairs of vertebra together is a concatenated chain of elements. Once the angular excursion is specified for each axis of rotation in each joint the model computes the configuration of the neck and draws a three-dimensional image that can be rotated in any direction to give different viewpoints for visual inspection. Measurements can also be collected directly from the calculation. Consequently, we can watch the neck move from any convenient perspective and plot the changes in selected parameters. An example of the use of calculated parameters to analyze the motion of the cervical spine is illustrated above. The drawn vertebrae represent the configuration of the neck in neutral position, fully flexed, and fully extended. The neck was flexed forward and extended back according to physiological angular excursions and the centers of rotation for those movements. The general configurations of these artificial necks seem to be reasonable matches to actual necks. They give the correct ranges of motion for the head relative the thorax and divide the motion between the vertebrae in an appropriate manner. The main reason for constructing such a model is to address questions related to the anatomy of the lower cervical spine. More generally, such a model allows one to study the behavior of assemblages of bones. In the following section, the concept of an equivalent compound movement is developed and a protocol for computing such movements is derived. With that tool in hand we address the equivalent compound movements of the lower cervical spine, primarily in terms of finding the centers of rotation for the cervical vertebrae as the neck experiences various movements, such as flexion and extension, oblique rotation, and circumduction.
Bogduk, N. (1999). "The neck." Baillieres Best Pract Res Clin Rheumatol 13 Bogduk, N. and S. Mercer (2000). "Biomechanics of the cervical spine. I: Normal kinematics." Clin Biomech (Bristol, Avon) 15 Kapandji, I. A. (1974). The Physiology of the Joints. Annotated diagrams of the mechanics of the human joints. New York, Churchill Livingstone. Nordin, M. and V. H. Frankel (1989). Basic Biomechanics of the Musculoskeletal System. Philadelphia, Lea & Febiger.
|