Spinal Dynamics V: Another Oblique Rotation One of the advantages of mathematical models is that one can ask ’what if?’ questions. In this chapter we will briefly consider a consequences of a second oblique axis of rotation. If one looks at the anatomy of the lower cervical spine, it appears that there is another possibility for an oblique movement, about an axis at a right angle to the usual axis. The principal restraints upon anatomical movements are impingements and tetherings, which are mediated by ligaments and bone configurations. Bones cannot move where they would overlap, that is, impinge. More generally, bones may be separated by cartilage or ligaments that becomes compressed between the bones and prevent them moving closer. In the latter case, the impingement is usually softer in that there is some play. Of course, cartilage is usually located where it is to allow controlled compression of the space between bones as well as to provide a lubricated surface. Ligaments and, to a lesser extent, joint capsules are generally placed so that they can prevent the separation of bones beyond a certain distance. They tether the bones. Both impingements and tethering may constrain movement and act as fulcra for rotations (Langer 2005g). The constraints upon the movements of the lower cervical vertebrae are the facet joints that act principally through impingement and the intervertebral discs, which act principally through tethering. The intervertebral discs are important support elements in that they separate the vertebrae and resist compression beyond a certain point, but it is their fibrocartilaginous portions that are the principal constraints upon rotations. In the lumbar spine, it is the helical coiling of the fibrous elements in the annulus fibrosus that allow the vertebra to rock into flexion and extension.
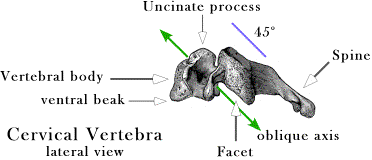
Cervical vertebrae mature by developing uncinate processes along the lateral margins of the rostral surface of the vertebral body and a bony beak along the ventral margin of the inferior surface. There are compensatory changes in the opposing surfaces so that the lateral margin of the inferior surface becomes slightly eroded and a facet surface forms and the ventral margin of the superior surface becomes modestly eroded and rounded. The intervertebral discs in the lower cervical spine are constructed quite differently than the pattern that is seen in the lumbar spine (Bogduk 1999; Bogduk and Mercer 2000; Levangie and Norkin 2001). Apparently, the cervical discs start out looking much like the intervertebral discs in the lumbar spine, with an annular fibrous ring around a central nucleus pulposus, but as the individual matures the configuration changes. In young spines, there are no uncinate processes rising at the lateral margins of the vertebral body. Probably starting in the second decade, the vertebrae and their discs begin to change. The posterior half of the lateral margin of the vertebral body develops a rostral flange and a caudal erosion, so that the joint is a bit more tubular. The rostral vertebra is cupped in the uncinate processes of the caudal vertebra in such a way as to encourage lateral rocking, but to discourage lateral rotation. However, the intervertebral discs are unusually thick in the neck so the vertebrae are not deeply seated and the constraint is not severe. The ventral part of the inferior margin of the cervical vertebrae becomes caudally extruded, to form a beak-like process that overhangs the caudal vertebra, which may becomes a bit eroded on its ventral dorsal margin. About the same time that the uncinate processes develop, the intervertebral discs also undergo a number of changes. The annulus fibrosus recedes until it is a crescent-shaped band along the ventral margin of the vertebral body, thickest in the midline and thinning to a thin band as it meets the uncinate process (anterior annular ligament). The fibers in the crescent are inclined so that they run rostrally and medially as they run rostrally. There is also a thin band in the dorsal midline (posterior annular ligament). The fibers in the ligament run longitudinally. The nucleus pulposus becomes larger and firmer, like a hard soap (fibrocartilaginous disc). In addition, it tends to develop horizontal fissures laterally that may extend substantial distances into the disc, frequently across the entire width. The lateral margins of the disc tend to end short of the uncinate processes and the space between fills with a lubricating fluid, creating a synovial joint.
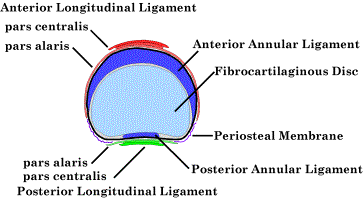
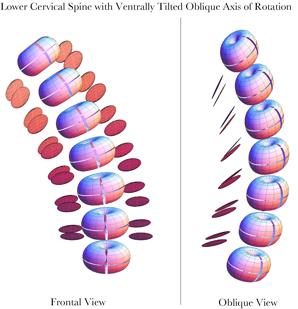
Diagram of a typical cervical intervertebral disc in a mature spine, based on the descriptions of Bogduk and Mercer. The annulus fibrous is a crescent in the ventral margin of the disc and a narrow band in the dorsal midline. Both components are reinforced by ligaments, the anterior and posterior longitudinal ligaments. The uncinate processes lie lateral to the dorsal part of the disc. It would appear that the joint between the vertebral bodies allows a significant amount of rotation about an axis that passes obliquely through the disc at a 45° angle. Such a movement is necessary to achieve the oblique movements that are normally ascribed to the lower cervical spine. In the case of the usual oblique movements, the axis of rotation is inclined so that the rostral end is tilted dorsally, perpendicular to the plane of the facet joints. Such movements are well accepted and they have been treated in considerable detail in earlier chapters. Now we come to the ‘what if?’ question. There is another possible axis of rotation allowed by the anatomy. It is indicated in the first figure above as a double-headed green arrow. If the axis passes through the center of the intervertebral disc parallel to the plane of the facet joints, then the strains in the disc and the ligaments would be comparable to the usual oblique movements and it would be much more compatible with the uncinate processes. Instead of being a twisting movement within the disc and its ligaments, it would be a rocking movement in which one uncinate joint would open slightly and the other would close an equal amount. The cupping of the uncinate processes would guide and restrain the movement. The pivot points in the annular ligaments will be in the midline and they will be such that one gets the maximal movement for a given angular excursion. The lateral wings of the anterior annular ligament will be inclined relative to the direction of movement, therefore able to accommodate the lateral opening of the joint more readily. The facet joints will be tangent to the movement, so that both joints move to the left or the right. There may be some compression and distraction in the facet joints, but given the angular excursions and the tangential disposition of the facets, it is entirely possible for the movement to occur without bony impingement. All of this argues that the movement is credible. With the model one can look at the implications of such a movement in a lower cervical spine. If we assume the same artificial lower cervical spine as in the previous chapters and an oblique axis of rotation that passes through the center of the intervertebral disc, tilted 45° anteriorly, then the configuration of the spine can be computed in the same way as for all the other examples. In the model, it requires changing one negative sign to a positive sign. The excursions are assumed to be as before, 10° in the joints above and below C5, 7.5° in the next joints in both directions, and 5° in the most rostral and most caudal joints. If viewed from the front (left panel in the following figure) positive rotation causes the spine to be sideflexed to the right and laterally rotated to the left. The directions of the two component movements have the same sign (positive in both cases). If the neck is bent to the right, the head ends up looking up and to the left. This is the opposite of the other oblique axis of rotation, where the component rotations are of opposite sign and the head ends up down to the same side as the neck is sideflexed. What is more critical here is the movements of the facets. The facets opposite to the direction of sideflexion are opened by the oblique movement and those on the same side are closed. It is difficult to tell how much impingement there may be from the frontal view, except that the facets do not penetrate each other. If the spine is turned 45° to the left (right panel of the following figure), then the facets that are coming together are seen in profile. The opposite facets are hidden behind the vertebral bodies. It can be readily seen that the facets do come close to impingement where the rotational excursions are 10°, that is in the middle joints. At 7.5° and 5° of rotation, there is quite adequate space between the facets. When viewed from other perspectives it is clear that the limits are apt to be impingement of the lateral margins of the closing facets and the stretching of the joint capsule for the opening facets.
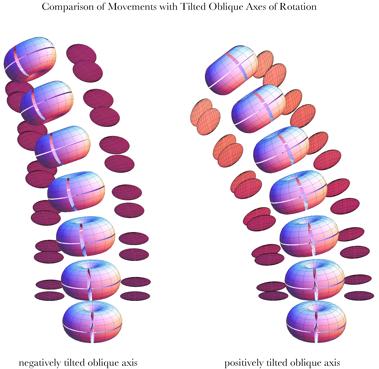
The artificial lower cervical spine was computed for rotations about a ventrally tilted oblique axis of rotation. The angular excursions are (5°, 7.5°, 10°, 10°, 7.5°, 5°) for the successive intervertebral joints. The same spine is shown in both panels. The spine in the right panel has been rotated 45° to the left relative to the spine in the left panel. Although this type of rotation is not described in the kinesiology literature, it would appear that it is anatomically possible. It does not strain the ligaments any more than the dorsally tilted axis and it is not limited by facet impingement. When combined with flexion, the impingement is reduced. When combined with extension, there is an intersection of the facets in the joints that experience both 10° of oblique rotation and 10° of extension, but not when the values are 7.5° for both rotations. Consequently, extension will be limited with oblique rotation if the axis of rotation is tilted ventrally relative to the vertebra. Whether or not oblique rotations about ventrally tilted axes actually occur, these calculations give a very nice illustration of the relationship between the axis of rotation and the frame of the object that is rotating. When the axis is tilted negatively relative to the frame the signs of the component movements about the frame vectors are opposite. That is somewhat confusing because the usual conventions would have them going in the same direction, that is, sideflexion and lateral rotation both to the right or both to the left. When the axis of rotation is tilted positively, the signs of the movements are the same. Unfortunately, that means that they are opposite in the usual naming convention. If the axis is aligned with one of the frame vectors, then there is no coupling between the movements. If it is directly rostrocaudal, then the rotations are lateral rotation alone, and if it is dorsoventral, the movements are sideflexion alone.
The same artificial lower cervical spine is rotated about a 45° negatively tilted axis of rotation and a 45° positively tilted axis of rotation. Note the relationships between the directions of sideflexion and lateral rotation. Bogduk, N. (1999). "The neck." Baillieres Best Pract Res Clin Rheumatol 13 Bogduk, N. and S. Mercer (2000). "Biomechanics of the cervical spine. I: Normal kinematics." Clin Biomech (Bristol, Avon) 15 Levangie, P. K. and C. C. Norkin (2001). Joint Structure and Function. A Comphrehensive Analysis. Philadelphia, F. A. Davis Company. |